AN50 APPLICATION NOTE
12
Embedded Sense Resistor (PC Trace Resistor)
Embedded PC trace resistors have the advantage of near zero
cost implementation. However, the value of the PC trace
resistor has large variations. Embedded resistors have 3
major error sources: the sheet resistivity of the inner layer,
the mismatch due to L/W, and the temperature variation of
the resistor. All three error sources must be considered for
laying out embedded sense resistors.
• Sheet resistivity.
For 1 ounce copper, the thickness variation is typically
1.15 mil to 1.35 mil. Therefore error due to sheet resistiv-
ity is (1.35 - 1.15)/1.25 = 16%
• Mismatch due to L/W.
Percent error in L/W is dictated by geometry and the
power dissipation capability of the sense resistor. The
sense resistor must be able to handle the load current and
therefore requires a minimum width which is calculated as
follows.
where: W = minimum width required for proper power
dissipation (mils) and I
L
= Load Current in Amps.
For 15A of load current, minimum width required is
300mils, which reflects a 1% L/W error.
• Thermal Consideration.
Due to I
2
R power losses the surface temperature of the
resistor will increase leading to a higher value. In addition,
ambient temperature
variation will add the change in resistor value:
where: R
20
is the resistance at 20°C,
α
20
= 0.00393/ °C, T
is the operating temperature, and R is the desired value.
For temperature T = 50°C, the %R change = 12%.
Table 9 is the summary of the tolerance for the Embedded
PC Trace Resistor.
Table 9. Summary PC Trace Resistor Tolerance
Design Rules for Using an Embedded Resistor
The basic equation for laying an embedded resistor is:
where:
ρ = Resistivity(µΩ-mil),
L = Length(mils),
W = Width(mils), and
t = Thickness(mils).
For 1oz copper, t = 1.35 mils, ρ = 717.86 µΩ-mil,
1 L/1 W = 1 Square ( ■ ).
For example, you can layout a 5.30mΩ embedded sense
resistor using the equations above:
L/W = 10 ■
Therefore, to model 5.30mΩ embedded sense resistor, you
need W = 200 mils and L = 2000 mils. Refer to Figure 9.
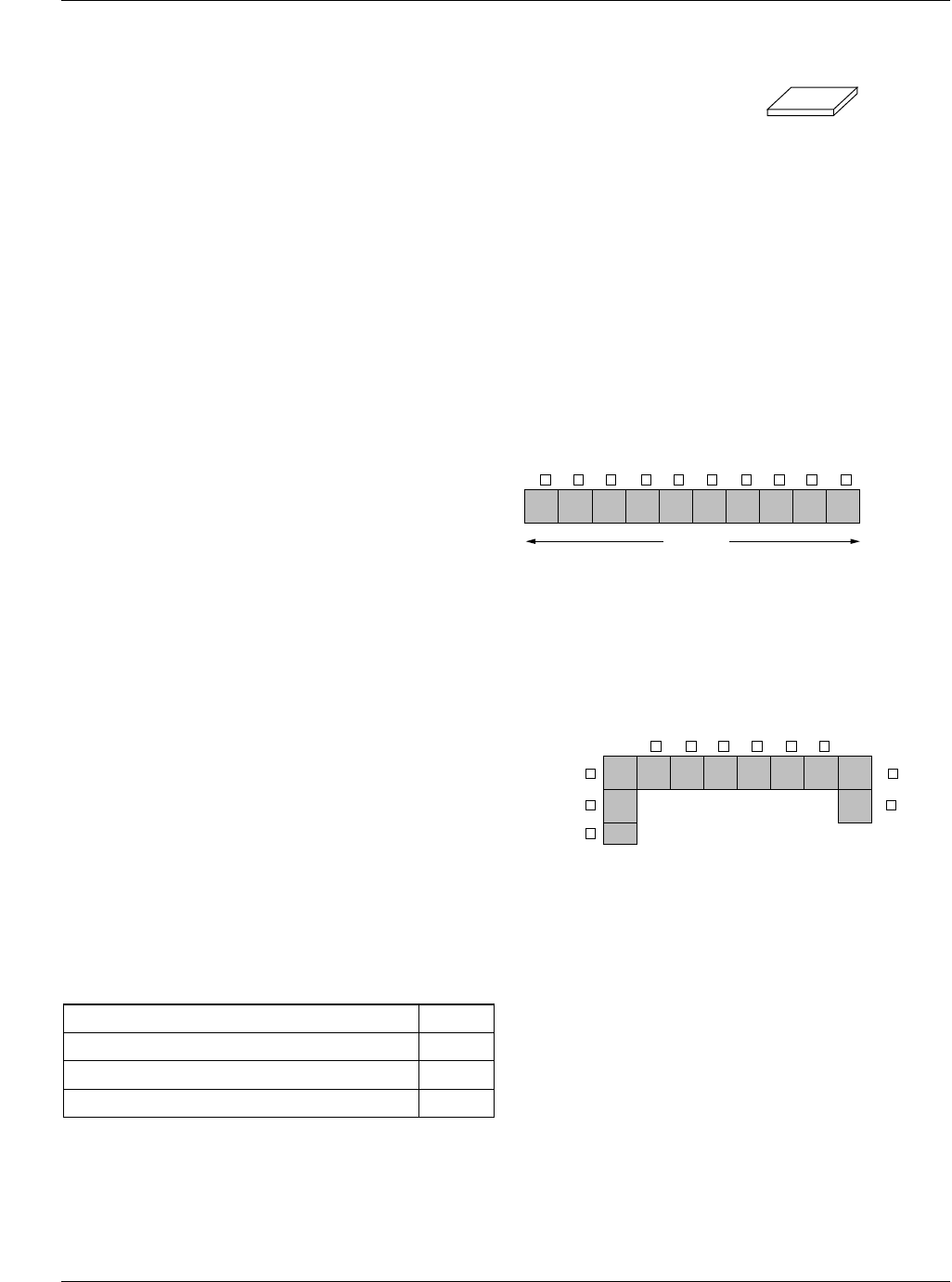
Figure 9. 5.30mΩ Sense Resistor (10 ■)
You can also implement the sense resistor in the following
manner. Each corner square is counted as 0.6 square since
current flowing through the corner square does not flow
uniformly and it is concentrated towards the inside edge, as
shown in Figure 10.
Figure 10. 5.30mΩ Sense Resistor (10 ■)
A Design Example Combining an Embedded Resistor
and a Discrete Resistor
For low cost implementation, the embedded PC trace resistor
is most desirable. However, its wide tolerance (29%) pre-
sents a challenge. In addition, requirements for the CPU
change frequently, and, thus, the maximum load current may
be subject to change. Combining embedded resistors with
discrete resistors may be a desirable option. Figure 11 shows
a design that provides flexibility with a solution to address
wide tolerances.
In this design, you have the option to choose an embedded
or a discrete MnCu sense resistor. To use the discrete sense
resistor, populate R21 with a shorting bar (zero Ohm resis-
tor) for proper Kelvin connection and add the MnCu sense
resistor. To use the embedded sense resistor, on the other
hand, populate R22 with a shorting bar for Kelvin connec-
Tolerance due to Sheet Resistivity variation 16%
Tolerance due to L/W error 1%
Tolerance due to temperature variation 12%
Total Tolerance for PC Trace Resistor 29%
W
I
L
0.05
----------=
R R
20
1 α
20
T 20–( )]+[=
R ρ
L
W t
×
-------------
×=
W
I
L
0.05
----------
10
0.05
---------- 200mils= = =
L
R W× t×
ρ
------------------------
0.00530 200× 1.35×
717.86
--------------------------------------------------- 2000mils= = =
1 1 1 1 1 1 1 1 1 1
W = 200 mils
L = 2000
.6 .6
.8
1 1 1 1 1 1
1 1
W
L
t


















