© Texas Instruments, 2007
Trigonometry with the TI-84 Plus Silver Edition
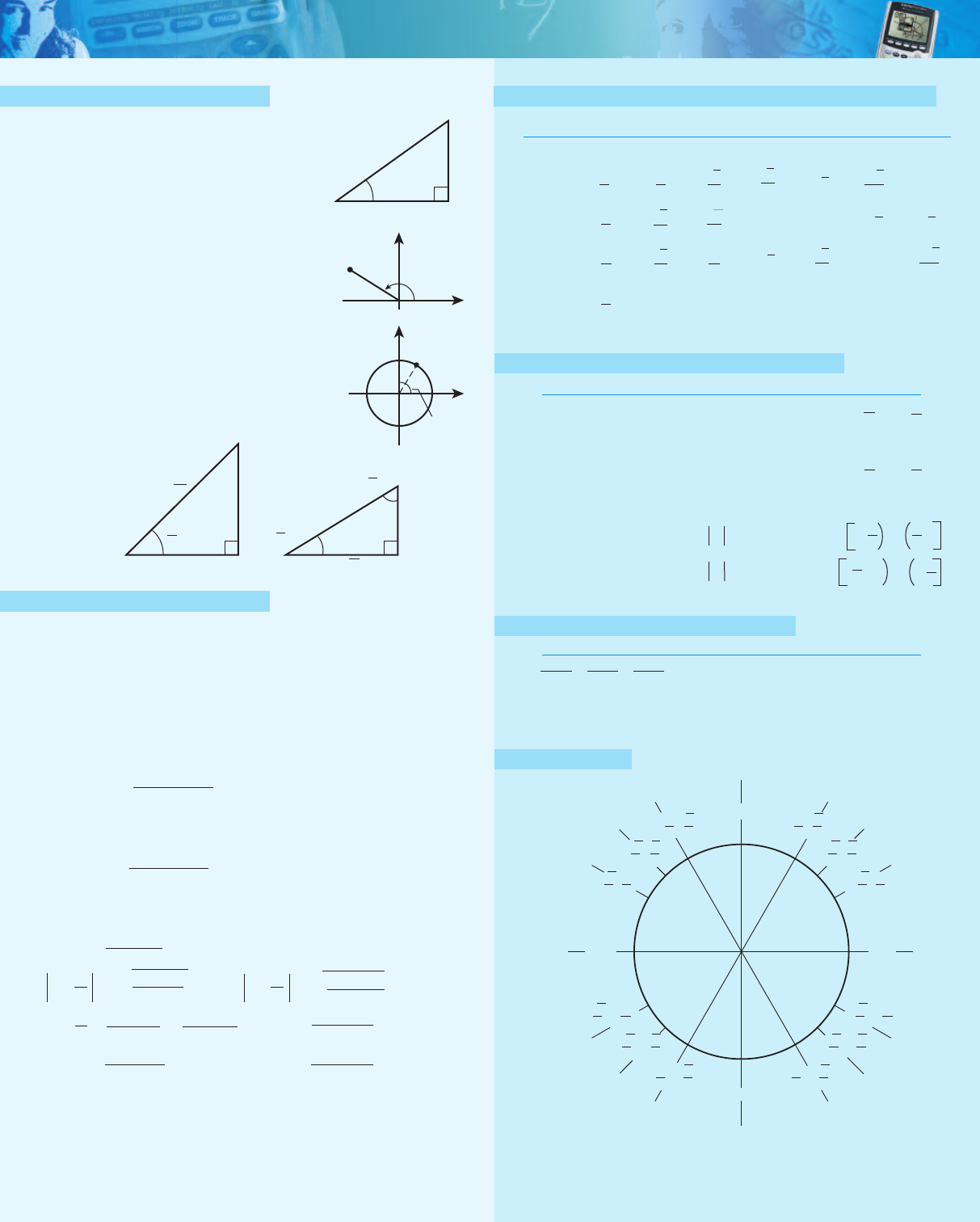
Trigonometric Functions
Acute Angles
ò sin
=
opp
/
hyp
csc
=
hyp
/
opp
ô cos
=
adj
/
hyp
sec
=
hyp
/
adj
ö tan
=
opp
/
adj
cot
=
adj
/
opp
Arbitrary Angles
sin
=
b
/
r
csc
=
r
/
b
cos
=
a
/
r
sec
=
r
/
a
tan
=
b
/
a
cot
=
a
/
b
Real Numbers
sin t = y csc t =
1
/
y
cos t = x sec t =
1
/
x
tan t =
y
/
x
cot t =
x
/
y
Special Triangles
Trigonometric Identities
csc t = 1/sin t tan t = sin t/cos t
sec t = 1/cos t cot t = cos t/sin t
cot t = 1/tan t
sin
2
t + cos
2
t = 1 sin (–t) = –sin t
1 + tan
2
t = sec
2
t cos (–t) = cos t
1 + cot
2
t = csc
2
t tan (–t) = –tan t
sin (u + v) = sin u cos v + cos u sin v
cos (u + v) = cos u cos v – sin u sin v
tan (u + v) =
sin (u – v) = sin u cos v – cos u sin v
cos (u – v) = cos u cos v + sin u sin v
tan (u – v) =
sin 2u =2 sin u cos u
cos 2u = cos
2
u – sin
2
u = 1 – 2 sin
2
u = 2 cos
2
u – 1
tan 2u =
sin = cos =
tan = = tan
2
u =
sin
2
u = cos
2
u =
sin u cos v =
1
/
2
[sin (u + v) + sin (u – v)]
cos u sin v =
1
/
2
[sin (u + v) – sin (u – v)]
cos u cos v =
1
/
2
[cos (u + v) + cos (u – v)]
sin u sin v =
1
/
2
[cos (u – v) – cos (u + v)]
opp
y
r
(a,b)
x
adj
hyp
1
1
π
4
y
t
(1,0)
t radians
(x,y)
x
=45°
1
√3
π
6
2
40°
60°
60°
4
2
c
3
b
=30°
π
3
=60°
√2
90°
270°
300°240°
315°
225°
330° 210°
0°180°
30°150°
45°135°
60°120°
(0,1)
(1,0)
(0,-1)
(-1,0)
(
,
)
1 √3
22
(
,
)
1 √3
22
(
, -
)
1 √3
22
(
, -
)
1 √3
22
(
,
)
√2 √2
22
(
,
-
-
)
√2 √2
22
(
, -
)
√2 √2
22
(
, -
)
√2 √2
22
(
,
)
√31
22
(
,-
)
√31
22
(
, -
)
√31
22
(
, -
-
-
-
)
√31
22
opp
y
r
(a,b)
x
adj
hyp
1
1
π
4
y
t
(1,0)
t radians
(x,y)
x
=45°
1
√3
π
6
2
40°
60°
60°
4
2
c
3
b
=30°
π
3
=60°
√2
90°
270°
300°240°
315°
225°
330° 210°
0°180°
30°150°
45°135°
60°120°
(0,1)
(1,0)
(0,-1)
(-1,0)
(
,
)
1 √3
22
(
,
)
1 √3
22
(
, -
)
1 √3
22
(
, -
)
1 √3
22
(
,
)
√2 √2
22
(
,
-
-
)
√2 √2
22
(
, -
)
√2 √2
22
(
, -
)
√2 √2
22
(
,
)
√31
22
(
,-
)
√31
22
(
, -
)
√31
22
(
, -
-
-
-
)
√31
22
opp
y
r
(a,b)
x
adj
hyp
1
1
π
4
y
t
(1,0)
t radians
(x,y)
x
=45°
1
√3
π
6
2
40°
60°
60°
4
2
c
3
b
=30°
π
3
=60°
√2
90°
270°
300°240°
315°
225°
330° 210°
0°180°
30°150°
45°135°
60°120°
(0,1)
(1,0)
(0,-1)
(-1,0)
(
,
)
1 √3
22
(
,
)
1 √3
22
(
, -
)
1 √3
22
(
, -
)
1 √3
22
(
,
)
√2 √2
22
(
,
-
-
)
√2 √2
22
(
, -
)
√2 √2
22
(
, -
)
√2 √2
22
(
,
)
√31
22
(
,-
)
√31
22
(
, -
)
√31
22
(
, -
-
-
-
)
√31
22
opp
y
r
(a,b)
x
adj
hyp
1
1
π
4
y
t
(1,0)
t radians
(x,y)
x
=45°
1
√3
π
6
2
40°
60°
60°
4
2
c
3
b
=30°
π
3
=60°
√2
90°
270°
300°240°
315°
225°
330° 210°
0°180°
30°150°
45°135°
60°120°
(0,1)
(1,0)
(0,-1)
(-1,0)
(
,
)
1 √3
22
(
,
)
1 √3
22
(
, -
)
1 √3
22
(
, -
)
1 √3
22
(
,
)
√2 √2
22
(
,
-
-
)
√2 √2
22
(
, -
)
√2 √2
22
(
, -
)
√2 √2
22
(
,
)
√31
22
(
,-
)
√31
22
(
, -
)
√31
22
(
, -
-
-
-
)
√31
22
tan u + tan v
1 – tan u tan v
tan u – tan v
1 + tan u tan v
2 tan u
1 – tan
2
u
1 – cos u
sin u
sin u
1 + cos u
u
2
1 – cos 2u
2
1 + cos 2u
2
u
2
1 – cos u
2
u
2
1 + cos u
2
√
√
Special Values of Trigonometric Functions
(
degrees) (radians)
sin
cos
tan
cot
sec
csc
0
° 0010–1 –
30° 2
4
5° 11
6
0° 2
90° 10–0–1
π
6
1
2
√3
2
√
3
3
√3
2√3
3
π
4
√
2
2
√
2
2
√
2 √2
√
3
2
1
2
√
3
√3
3
2√3
3
π
2
Laws of Sines and Cosines
Sine Cosine
sin A = sin B = sin C a
2
= b
2
+ c
2
– 2bc cos A
a b c
c
2
= a
2
+ b
2
– 2ab cos C
b
2
= c
2
+ a
2
– 2ac cos B
Unit Circle
opp
y
r
(a,b)
x
adj
hyp
1
1
π
4
y
t
(1,0)
t radians
(x,y)
x
=45°
1
√3
π
6
2
40°
60°
60°
4
2
c
3
b
=30°
π
3
=60°
√2
90°
270°
300°240°
315°
225°
330° 210°
0°180°
30°150°
45°135°
60°
120°
(0,1)
(1,0)
(0,-1)
(-1,0)
(
,
)
1 √3
2
2
(
,
)
1 √3
2
2
(
, -
)
1 √3
2
2
(
, -
)
1 √3
2
2
(
,
)
√2 √2
2
2
(
,
-
-
)
√2 √2
2
2
(
, -
)
√2 √2
2
2
(
, -
)
√2 √2
2
2
(
,
)
√31
2
2
(
,-
)
√31
2
2
(
, -
)
√31
2
2
(
, -
-
-
-
)
√31
2
2
π
3
Inverse Trigonometric Functions
Function Domain Range
y
? y = sin
-
1
x
–1
≤
x
≤
1 –
≤
y
≤
y @ y = cos
-1
x –1
≤
x
≤
1 0
≤
y
≤π
y A y = tan
-1
x All real numbers – < y <
y = cot
-1
x All real numbers 0 < y <
π
y = sec
-1
x x
≥
1 0, ∪
y = csc
-1
x x
≥
1 – , 0 ∪ 0,
π
2
π
2
π
2
π
2
π
2
π
2
π
2
education.ti.com
1 - cos 2u
1 + cos 2u
π
2
,
π












