EL-9650/9600c Graphing Calculator
There may be differences in the results of calculations and graph plotting depending on the setting.
Return all settings to the default value and delete all data.
NotesStep & Key Operation
*Use either pen touch or cursor to operate.
Display
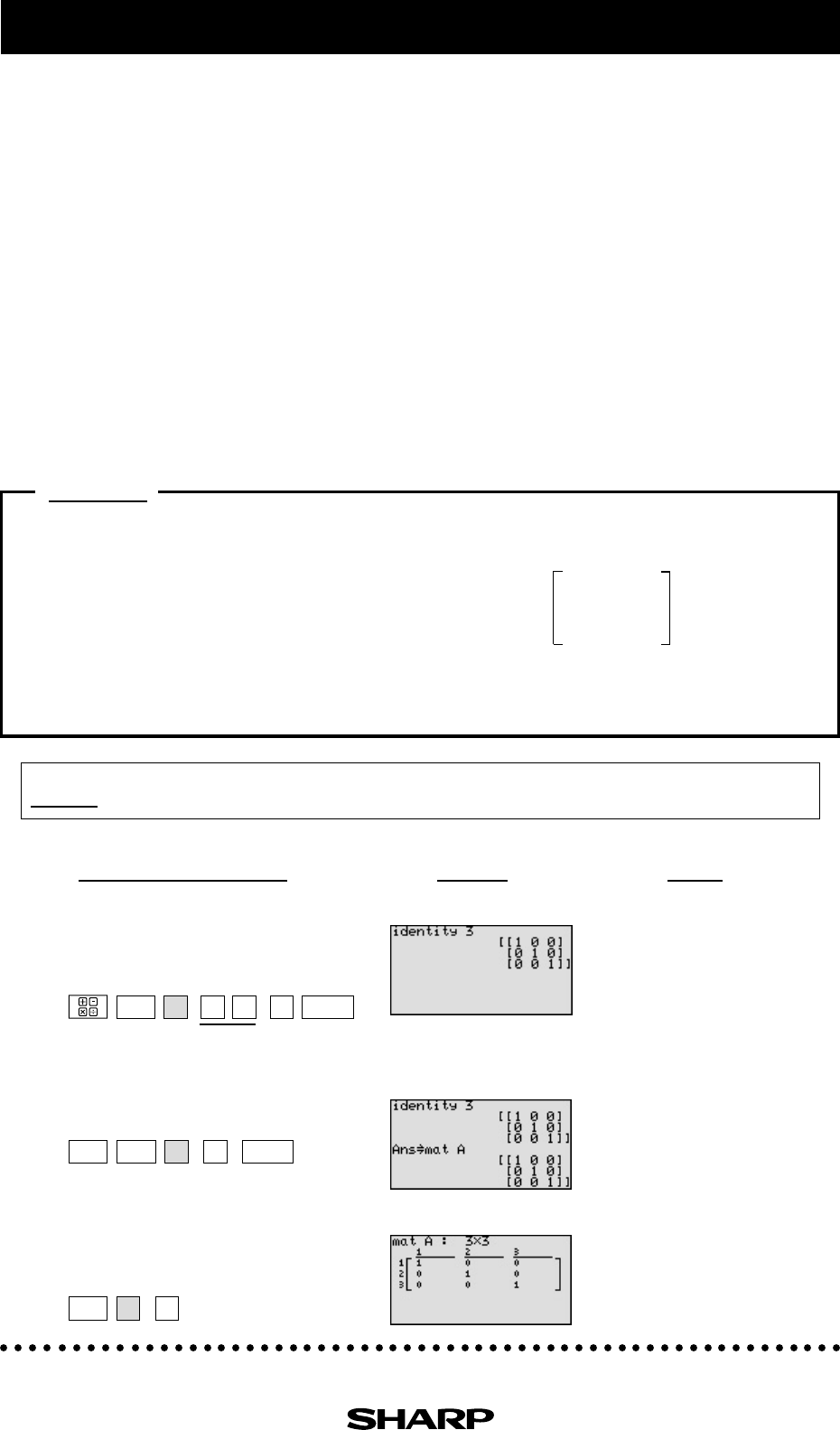
1. Enter the 3 x 3 identity matrix in matrix A.
2. Find the inverse matrix of the matrix B.
3. Solve the equation system.
x + 2y + z = 8
2x + y - z = 1
x + y - 2z = -3
Example
Use matrix multiplication to solve a system of linear equations.
B
1 2 1
2 1 -1
1 1 -2
Solving a System of Linear Equations Using Matrices
Set up 3 x 3 identity matrix at the
home screen.
Save the identity matrix in matrix A.
1-1
1-2
Confirm that the identity matrix is
stored in matrix A.
1-3
Each system of three linear equations consists of three variables. Equations in more than
three variables cannot be graphed on the graphing calculator. The solution of the system of
equations can be found numerically using the Matrix feature or the System solver in the
Tool feature.
A system of linear equations can be expressed as AX = B (A, X and B are matrices). The
solution matrix X is found by multiplying A
-1
B. Note that the multiplication is “order sensitive”
and the correct answer will be obtained by multiplying BA
-1
. An inverse matrix A
-1
is a
matrix that when multiplied by A results in the identity matrix I (A
-1
x A=I). The identity
matrix I is defined to be a square matrix (n x n) where each position on the diagonal is 1
and all others are 0.
6-2
*
*
*
*
*
*
{
MATRIX
MATRIX
MATRIX
05 3
A
1
B1
ENTER
ENTER
STO
C
Before
Starting
