20010101
uu
uu
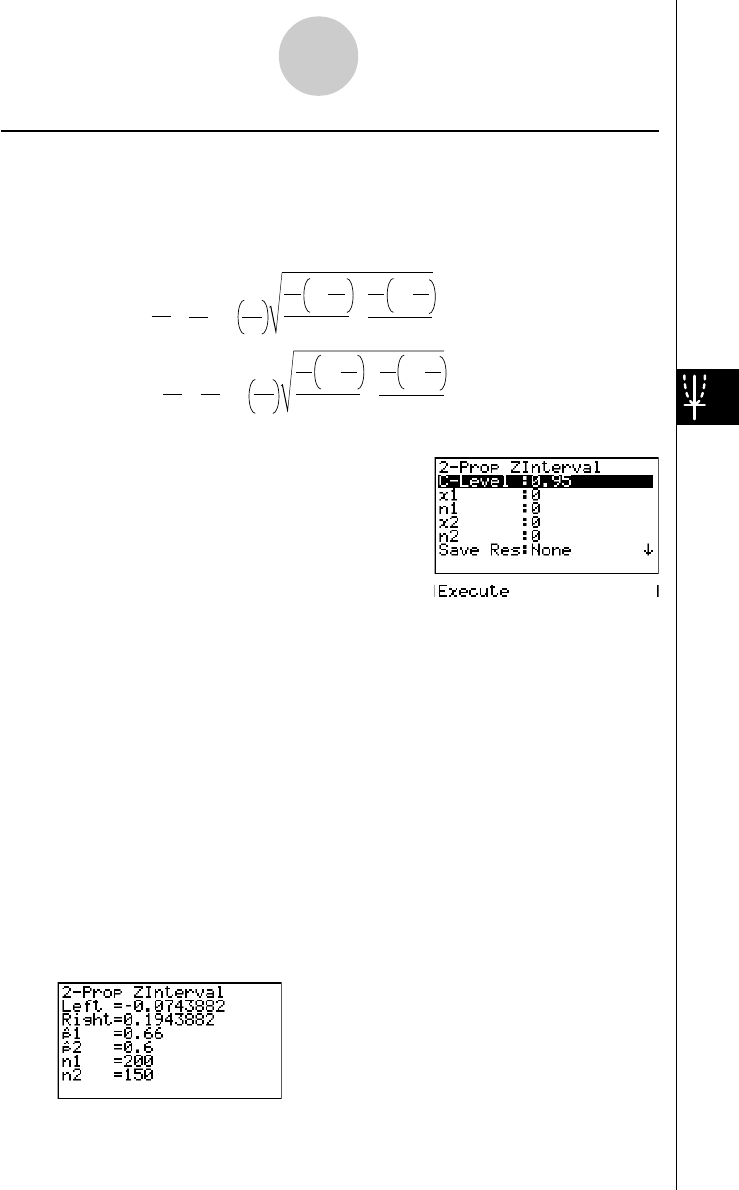
u 2-Prop Z Interval
2-Prop Z Interval uses the number of data items to calculate the confidence interval for the
defference between the proportion of successes in two populations.
The following is the confidence interval. The value 100 (1 –
α) % is the confidence level.
Left = – – Z
α
2
x
1
n
1
x
2
n
2
n
1
n
1
x
1
1–
n
1
x
1
+
n
2
n
2
x
2
1–
n
2
x
2
Right = – + Z
α
2
x
1
n
1
x
2
n
2
n
1
n
1
x
1
1–
n
1
x
1
+
n
2
n
2
x
2
1–
n
2
x
2
n1, n2 : size of sample
x1, x2 : data
Perform the following key operations from the statistical data list.
4(INTR)
b(Z)
e(2-Prop)
Data is specified using parameter specification. The following shows the meaning of each
item.
C-Level ........................ confidence level (0 < C-Level < 1)
x1 ................................. data value (x1 > 0) of sample 1
n1 ................................. size (positive integer) of sample 1
x2 ................................. data value (x2 > 0) of sample 2
n2 ................................. size (positive integer) of sample 2
Save Res ..................... list for storage of calculation results (None or List 1 to 20)
Execute ....................... executes a calculation
After setting all the parameters, align the cursor with [Execute] and then press the function
key shown below to perform the calculation.
• 1(CALC) ... Performs the calculation.
Calculation Result Output Example
1-3-7
Confidence Interval (INTR)
20011101


















