20020601
English
Activity: SetupActivity: Setup
2-8-1
í Equipment
Piano (Sound Source) Computer (MIDI Sound Source)
Audio Measurement Setup (EA-200, graphic scientific calculator, data communication
cable)
í Setting Up
u Take care so there is no unwanted noise in the area where you are conducting the activity.
The purpose of this activity is to investigate the scale that is most commonly used for
Western-style music, and to listen to some of the consonance it can produce.
The pitch of a note is determined by its frequency, and the human ear perceives notes as
differences in frequency ratios, rather than differences in the relative amplitude of the
frequency.
The ratios of the 12-note mean scale used for most Western-style music is governed by a
number of restrictions. First, the frequency ratio of the same note from one octave to the
next is 2:1 (higher note to lower note). Each octave is divided into 12 parts, with the same
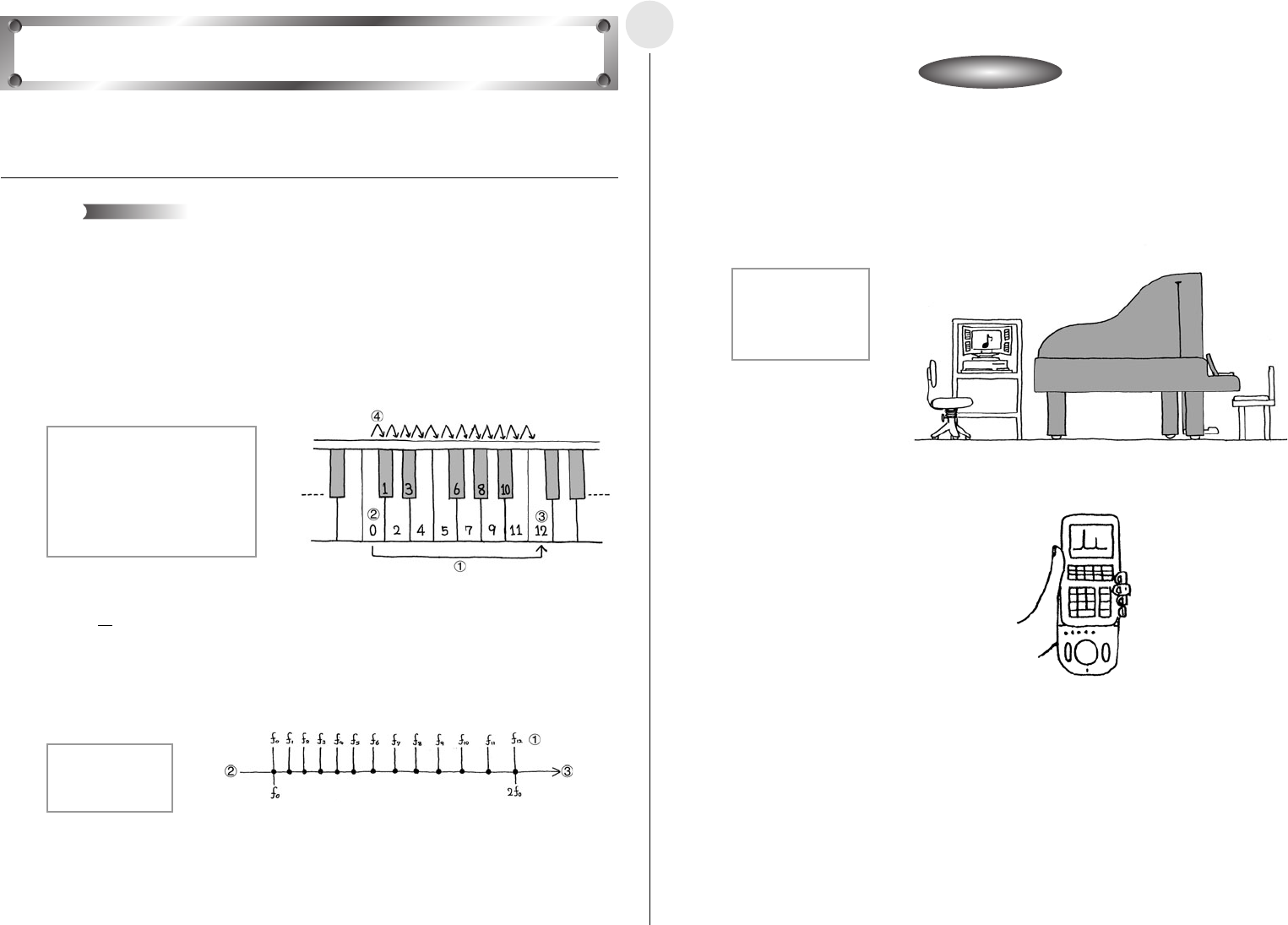
frequency ratio between each of the adjoining notes in the octave. The illustration below
shows each of the notes in an octave, on a piano keyboard.
The frequencies of this 12-note scale can be expressed as shown below.
f
n
= 2 f
0
f
0
(Hz) : Tonic Note Frequency
f
12
(Hz): Harmonic Frequency
f
n
(Hz) : Frequency of nth Note (n = 1, 2, 3, ....12)
Generally, notes consist of sound waves of different frequencies and amplitudes. Producing
two notes of different pitches at the same time sounds pleasing to the human ear, and such
notes are said to be “consonant.” Two notes whose frequency ratio is the ratio of two simple
integers are very consonant.
Construction of the Musical Scale
Theory
1 Piano
2 Computer (MIDI
Sound Source)
3 EA-200
1 1 Octave (2:1 frequency ratio
between notes)
2 Tonic Note (Low Note)
3 Harmonic (High Note)
4 Adjacent Note (Note
Frequency Ratio = 2
(1/12)
)
1 Frequency (Hz)
2 Low Note
3 High Note
n
12
1
2
3


















