E-85
Example 2: The nearby data shows how the weight of a
newborn at various numbers of days after birth.
1
Obtain the regression formula and correlation coeffi cient
produced by linear regression of the data.
2
Obtain the regression formula and correlation coeffi cient
produced by logarithmic regression of the data.
3
Predict the weight 350 days after birth based on the
regression formula that best fi ts the trend of the data in
accordance with the regression results.
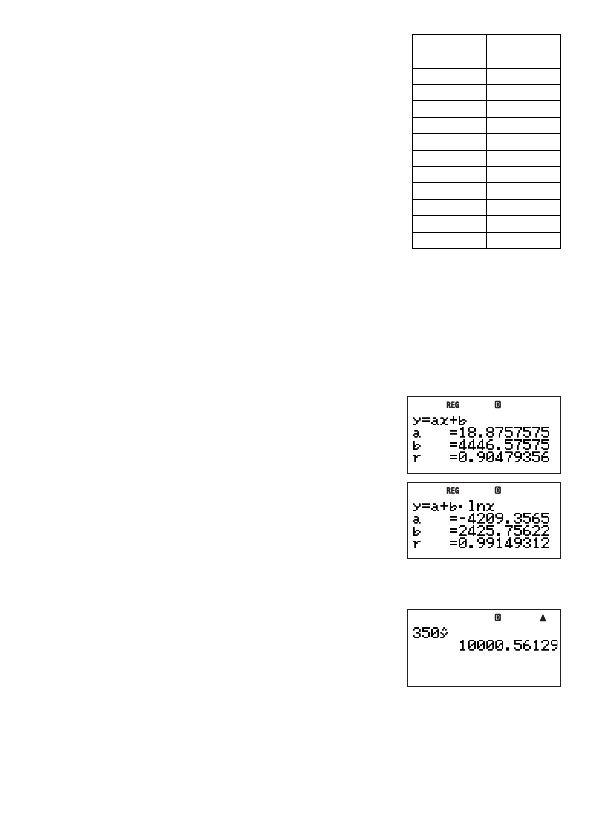
Number
of Days
Weight
(g)
20 3150
50 4800
80 6420
110 7310
140 7940
170 8690
200 8800
230 9130
260 9270
290 9310
320 9390
Operation Procedure
Select the REG Mode:
N
4
(REG)
Select FreqOff for the statistical frequency setting:
1N
(SETUP)
c
5
(STAT)
2
(FreqOff)
Input the number of day data into the X-column:
20
E
50
E
80
E
110
E
140
E
170
E
200
E
230
E
260
E
290
E
320
E
Input the weight data into the Y-column:
ce
3150
E
4800
E
6420
E
7310
E
7940
E
8690
E
8800
E
9130
E
9270
E
9310
E
9390
E
(1) Linear Regression
Display the linear regression calculation result screen:
z
6
(RESULT)
2
(Reg)
1
(Line)
(2) Distribution Logarithmic Regression
Display the logarithmic regression calculation result screen:
J
z
6
(RESULT)
2
(Reg)
3
(Log)
(3) Weight Prediction
The absolute value of correlation coeffi cient r is close to 1, so logarithmic regression is used
for its calculation.
Obtain
n
when
x
= 350:
J
z
1
(
/
COMP)
350
z
7
(STAT)
2
(VAR)
ccc
7
(
n
)
E
