20050401
uu
uu
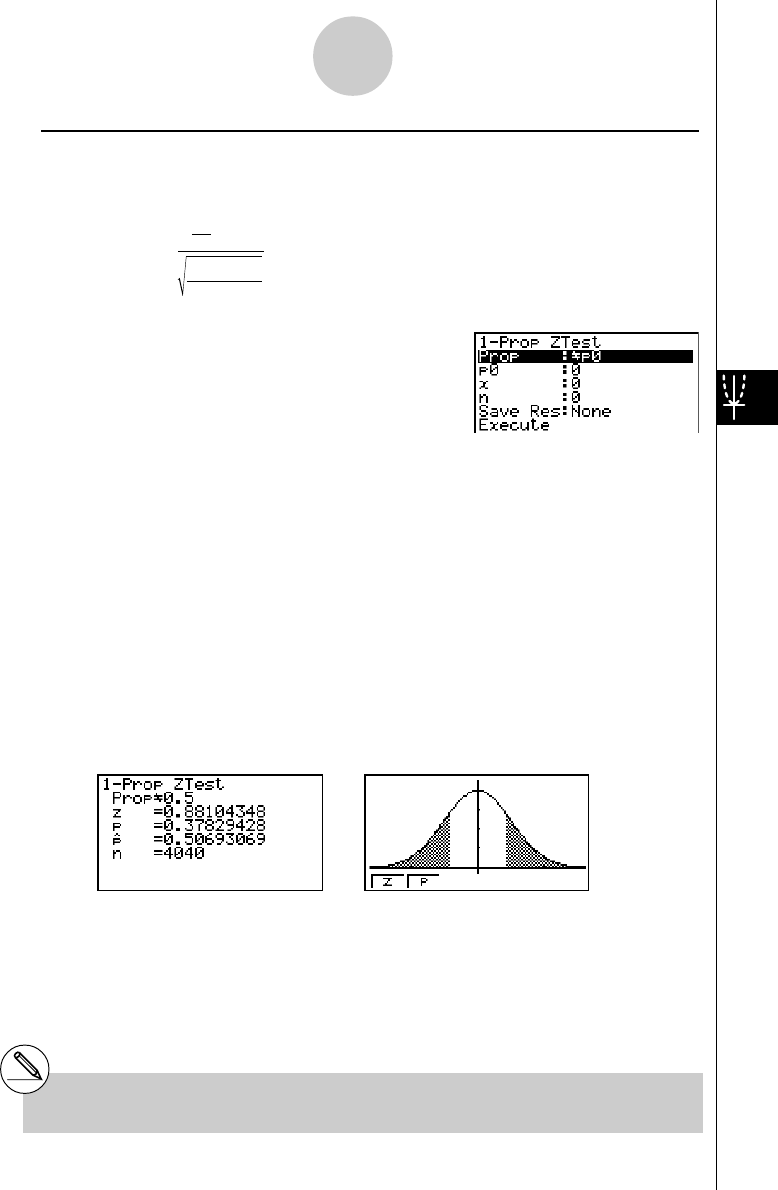
u 1-Prop
Z Test
This test is used to test for an unknown proportion of successes. The 1-Prop Z Test is
applied to the normal distribution.
Z =
n
x
n
p
0
(1– p
0
)
– p
0
p0 : expected sample proportion
n : size of sample
Perform the following key operations from the statistical data list.
3(TEST)
1(Z)
3(1-P)
Prop ............................ sample proportion test conditions (“G p0” specifies two-tail
test, “< p0” specifies lower one-tail test, “> p0” specifies upper
one-tail test.)
p0 ................................. expected sample proportion (0 < p0 < 1)
x .................................. sample value (x > 0 integer)
n .................................. size of sample (positive integer)
Save Res .................... list for storage of calculation results (None or List 1 to 26)
Execute ....................... executes a calculation or draws a graph
After setting all the parameters, use c to move the highlighting to “Execute” and then press
one of the function keys shown below to perform the calculation or draw the graph.
• 1(CALC) ... Performs the calculation.
• 6(DRAW) ... Draws the graph.
Calculation Result Output Example
PropG0.5 .................... direction of test
z ...................................z score
p ..................................p-value
ˆp ..................................estimated sample proportion
n ..................................size of sample
• For details about graph screen function keys 1(Z) and 2(P), see “Z Test
Common Functions” on page 6-5-2.
# [Save Res] does not save the Prop condition
in line 2.
6-5-7
Tests


















