Applications 17-17
8317APPS.DOC TI-83 international English Bob Fedorisko Revised: 02/19/01 1:00 PM Printed: 02/19/01 1:39 PM
Page 17 of 20
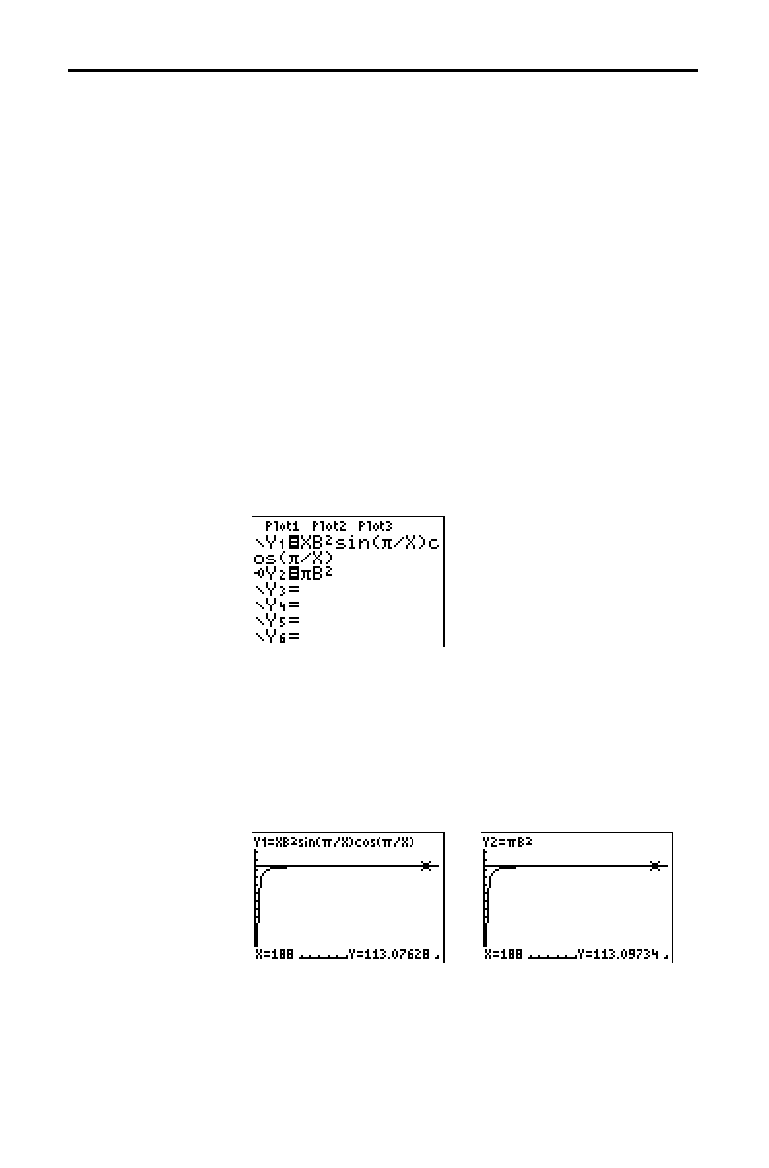
Find the area given B=6, and N=10, 100, 150, 1000, and
10000. Compare your results with
p
6
2
(the area of a circle
with radius 6), which is approximately 113.097.
7. Enter
B=6. To find the area A, move the cursor onto A,
and then press
ƒ
[
SOLVE
]. Find A for N=10, then
N=100, then N=150, then N=1000, and finally N=10000.
Notice that as
N gets large, the area A approaches
p
B
2
.
Now graph the equation to see visually how the area
changes as the number of sides gets large.
8. Press
z
. Select the default mode settings.
9. Press
p
. Set the viewing window.
Xmin=0 Ymin=0 Xres=1
Xmax=200 Ymax=150
Xscl=10 Yscl=10
10.Press
o
. Turn off all functions and stat plots. Enter the
equation for the area. Use
X in place of N. Set the graph
styles as shown.
11.Press
r
. After the graph is plotted, press 100
Í
to trace to
X=100. Press 150
Í
. Press 188
Í
.
Notice that as
X increases, the value of Y converges to
p
6
2
, which is approximately 113.097. Y
2
=
p
B
2
(the area of
the circle) is a horizontal asymptote to
Y
1
. The area of
an N-sided regular polygon, with r as the distance from
the center to a vertex, approaches the area of a circle
with radius r (
p
r
2
) as N gets large.


















