Filter
Top Products
609
Measures of Fit
This things-could-be-much-worse philosophy of model evaluation is incorporated into
a number of fit measures. All of the measures tend to range between 0 and 1, with
values close to 1 indicating a good fit. Only NFI (described below) is guaranteed to be
between 0 and 1, with 1 indicating a perfect fit. (CFI is also guaranteed to be between
0 and 1, but this is because values bigger than 1 are reported as 1, while values less than
0 are reported as 0.)
The independence model is only one example of a model that can be chosen as the
baseline model, although it is the one most often used and the one that Amos uses.
Sobel and Bohrnstedt (1985) contend that the choice of the independence model as a
baseline model is often inappropriate. They suggest alternatives, as did Bentler and
Bonett (1980), and give some examples to demonstrate the sensitivity of NFI to the
choice of baseline model.
NFI
The Bentler-Bonett (1980) normed fit index (NFI), or Δ
1
in the notation of Bollen
(1989b) can be written
where is the minimum discrepancy of the model being evaluated and
is the minimum discrepancy of the baseline model.
In Example 6, the independence model can be obtained by adding constraints to any
of the other models. Any model can be obtained by constraining the saturated model.
So Model A, for instance, with , is unambiguously in between the
perfectly fitting saturated model ( ) and the independence model
().
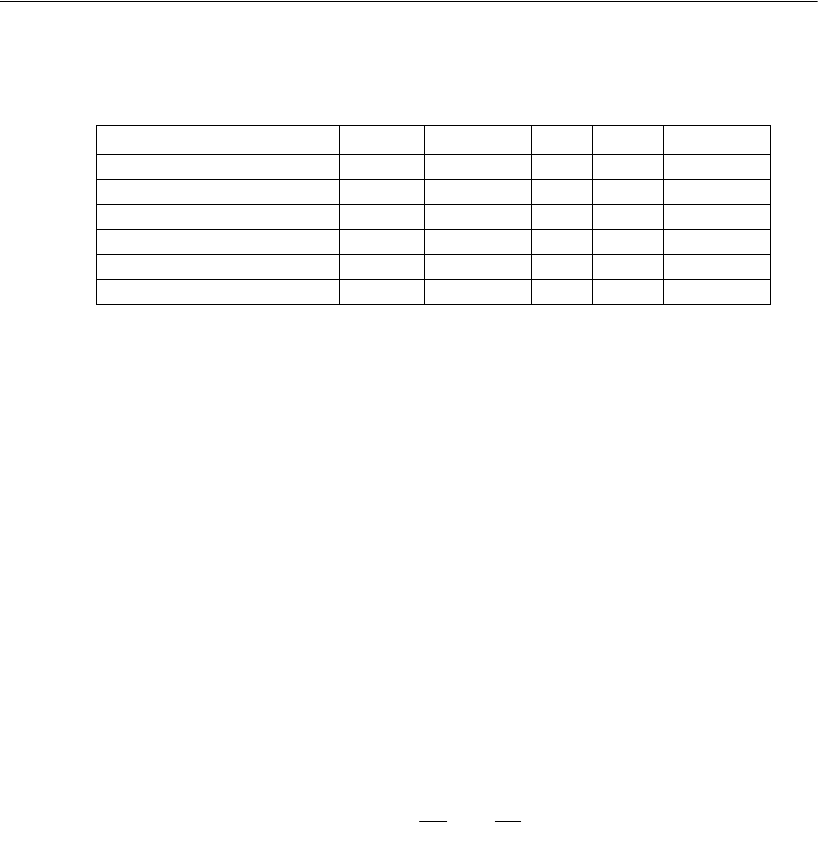
Model NPAR CMIN DF P CMIN/DF
Model A: No Autocorrelation 15 71.544 6 0.000 11.924
Model B: Most General 16 6.383 5 0.271 1.277
Model C: Time-Invariance 13 7.501 8 0.484 0.938
Model D: A and C Combined 12 73.077 9 0.000 8.120
Saturated model 21 0.000 0
Independence model 6 2131.790 15 0.000 142.119
b
b
F
F
C
C
ˆ
ˆ
1
ˆ
ˆ
1NFI
1
−=−=Δ=
C
ˆ
nF
ˆ
=
C
b
ˆ
nF
b
ˆ
=
χ
2
71.544=
χ
2
0=
χ
2
2131.790=