Page 14-5
A different version of the chain rule applies to the case in which z = f(x,y), x =
x(u,v), y = y(u,v), so that z = f[x(u,v), y(u,v)]. The following formulas represent
the chain rule for this situation:
Determining extrema in functions of two variables
In order for the function z = f(x,y) to have an extreme point (extrema) at (x
o
,y
o
),
its derivatives ∂f/∂x and ∂f/∂y must vanish at that point. These are necessary
conditions. The sufficient conditions for the function to have an extreme at point
(x
o
,y
o
) are ∂f/∂x = 0, ∂f/∂y = 0, and Δ = (∂
2
f/∂x
2
)⋅ (∂
2
f/∂y
2
)-[∂
2
f/∂x∂y]
2
> 0.
The point (x
o
,y
o
) is a relative maximum if ∂
2
f/∂x
2
< 0, or a relative minimum if
∂
2
f/∂x
2
> 0. The value Δ is referred to as the discriminant.
If Δ = (∂
2
f/∂x
2
)⋅ (∂
2
f/∂y
2
)-[∂
2
f/∂x∂y]
2
< 0, we have a condition known as a
saddle point, where the function would attain a maximum in x if we were to
hold y constant, while, at the same time, attaining a minimum if we were to
hold x constant, or vice versa.
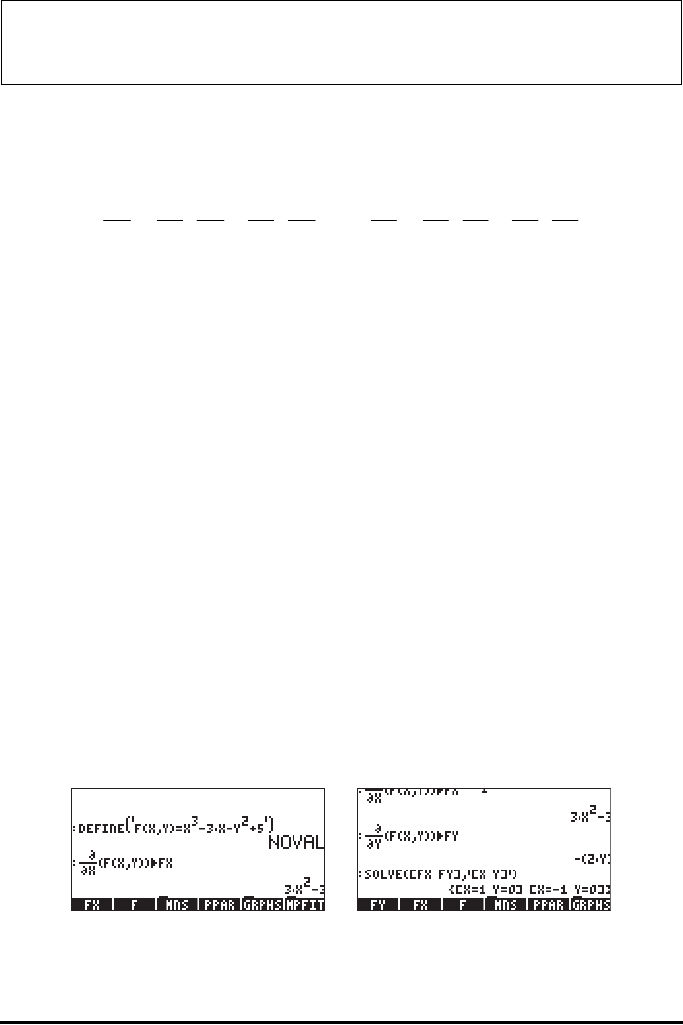
Example 1 – Determine the extreme points (if any) of the function f(X,Y) = X
3
-3X-
Y
2
+5. First, we define the function f(X,Y), and its derivatives fX(X,Y) = ∂f/∂X,
fY(X,Y) = ∂f/∂Y. Then, we solve the equations fX(X,Y) = 0 and fY(X,Y) = 0,
simultaneously:
Total differential of a function z = z(x,y)
From the last equation, if we multiply by dt, we get the total differential of the
function z = z(x,y), i.e., dz =
(∂z/∂x)⋅dx + (∂z/∂y)⋅dy.
v
y
y
z
v
x
x
z
v
z
u
y
y
z
u
x
x
z
u
z
∂
∂
⋅
∂
∂
+
∂
∂
⋅
∂
∂
=
∂
∂
∂
∂
⋅
∂
∂
+
∂
∂
⋅
∂
∂
=
∂
∂
,


















