Filter
Top Products
Page 16-62
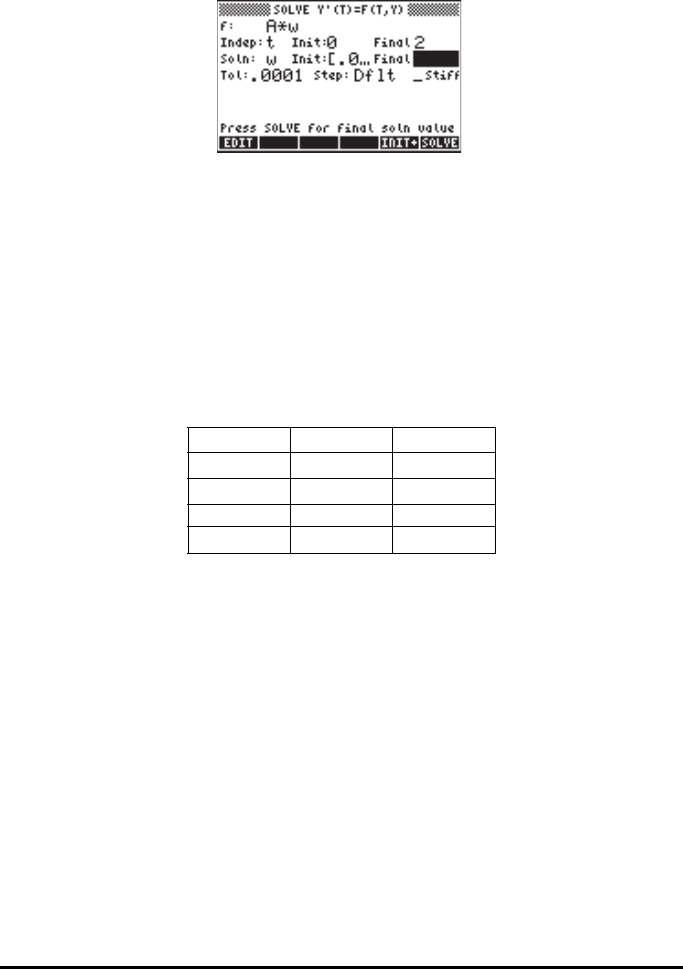
time t = 2, the input form for the differential equation solver should look as
follows (notice that the Init: value for the Soln: is a vector [0, 6]):
Press @SOLVE (wait) @EDIT to solve for w(t=2). The solution reads [.16716… -
.6271…], i.e., x(2) = 0.16716, and x'(2) = v(2) = -0.6271. Press @CANCL to
return to SOLVE environment.
Solution presented as a table of values
In the previous example we were interested only in finding the values of the
position and velocity at a given time t. If we wanted to produce a table of
values of x and x', for t = 0.00, 0.25, …, 2.00, we will proceed as follows:
First, prepare a table to write down your results:
Next, within the SOLVE environment, change the final value of the independent
variable to 0.25, use:
—.25 @@OK@@ ™™ @SOLVE (wait) @EDIT
(Solves for w at t = 0.25, w = [0.968 1.368]. )
@@OK@@ INIT+ — . 5 @@OK@@ ™™@SOLVE (wait) @EDIT
(Changes initial value of t to 0.25, and final value of t to 0.5, solve again for
w(0.5) = [0.748 -2.616])
@@OK@@ @INIT+ —.75 @@OK@@™™@SOLVE (wait) @EDIT
(Changes initial value of t to 0.5, and final value of t to 0.75, solve again for
w(0.75) = [0.0147 -2.859])
@@OK@@ @INIT+ —1 @@OK@@ ™ ™ @SOLVE (wait) @EDIT
txx'
0.00 0.00 6.00
0.25
………
2.00