Filter
Top Products
Page 16-56
Laguerre’s equation
Laguerre’s equation is the second-order, linear ODE of the form x⋅(d
2
y/dx
2
)
+(1−x)⋅ (dy/dx) + n⋅y = 0. Laguerre polynomials, defined as
,
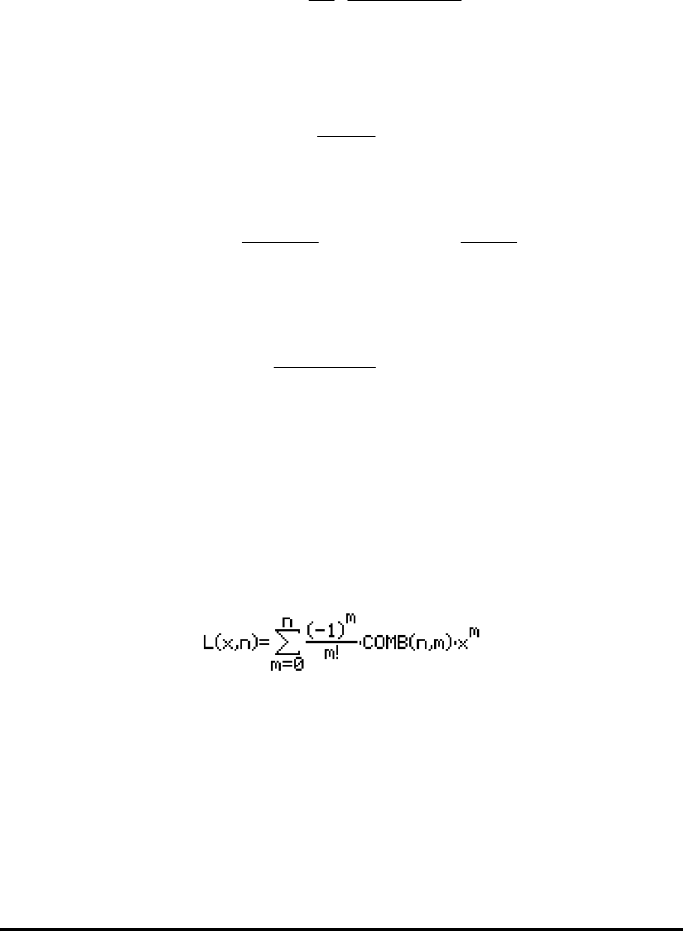
are solutions to Laguerre’s equation. Laguerre’s polynomials can also be
calculated with:
The term
is the m-th coefficient of the binomial expansion (x+y)
n
. It also represents the
number of combinations of n elements taken m at a time. This function is
available in the calculator as function COMB in the MTH/PROB menu (see also
Chapter 17).
You can define the following function to calculate Laguerre’s polynomials:
When done typing it in the equation writer press use function DEFINE to create
the function L(x,n) into variable @@@L@@@ .
To generate the first four Laguerre polynomials use, L(x,0), L(x,1), L(x,2), L(x,3).
The results are:
L
0
(x) = .
L
1
(x) = 1-x.
,...2,1,
)(
!
)(,1)(
0
=
⋅
⋅==
−
n
dx
exd
n
e
xLxL
n
xnnx
n
.
!
)1(
)(
0
m
n
m
m
n
x
m
n
m
xL ⋅
⎟
⎟
⎠
⎞
⎜
⎜
⎝
⎛
⋅
−
=
∑
=
n
n
x
n
x
nn
xn ⋅
−
++−⋅
−
+⋅−=
!
)1(
.......
4
)1(
1
2
),(
)!(!
!
mnC
mnm
n
m
n
=
−
=
⎟
⎟
⎠
⎞
⎜
⎜
⎝
⎛