Filter
Top Products
Page 16-27
The following exercises are in ALG mode, with CAS mode set to Exact. (When
you produce a graph, the CAS mode will be reset to Approx. Make sure to set
it back to Exact after producing the graph.) Suppose, for example, that the
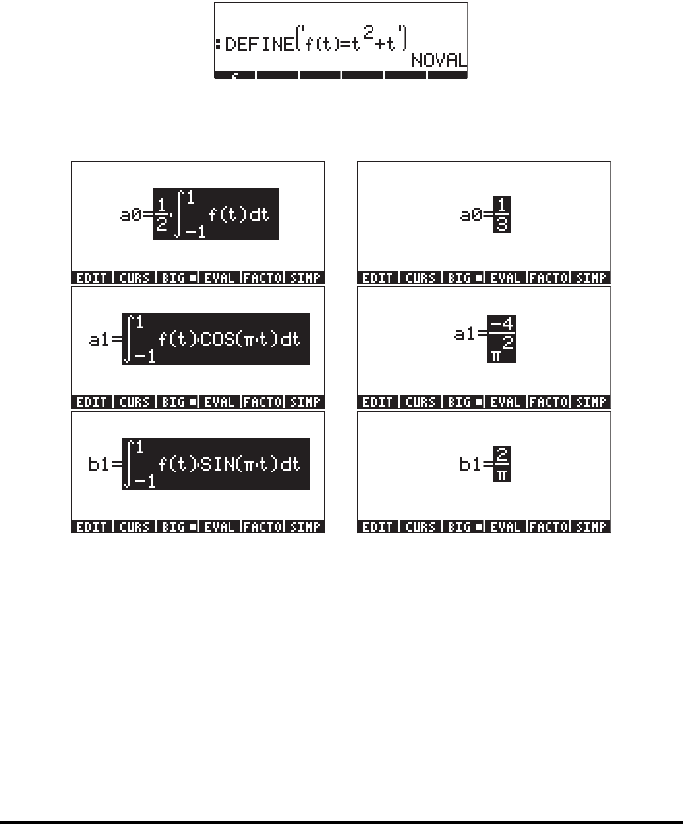
function f(t) = t
2
+t is periodic with period T = 2. To determine the coefficients
a
0
, a
1
, and b
1
for the corresponding Fourier series, we proceed as follows:
First, define function f(t) = t
2
+t :
Next, we’ll use the Equation Writer to calculate the coefficients:
Thus, the first three terms of the function are:
f(t) ≈ 1/3 – (4/π
2
)⋅cos (π⋅t)+(2/π)⋅sin (π⋅t).
A graphical comparison of the original function with the Fourier expansion
using these three terms shows that the fitting is acceptable for t < 1, or
thereabouts. But, then, again, we stipulated that T/2 = 1. Therefore, the fitting
is valid only between –1 < t < 1.